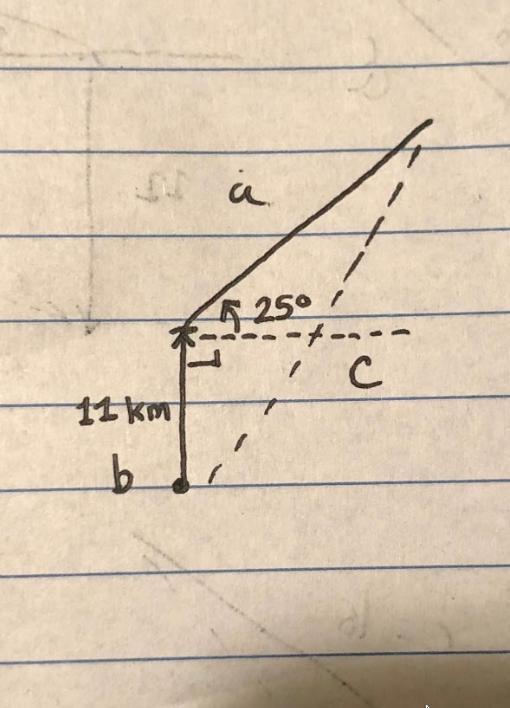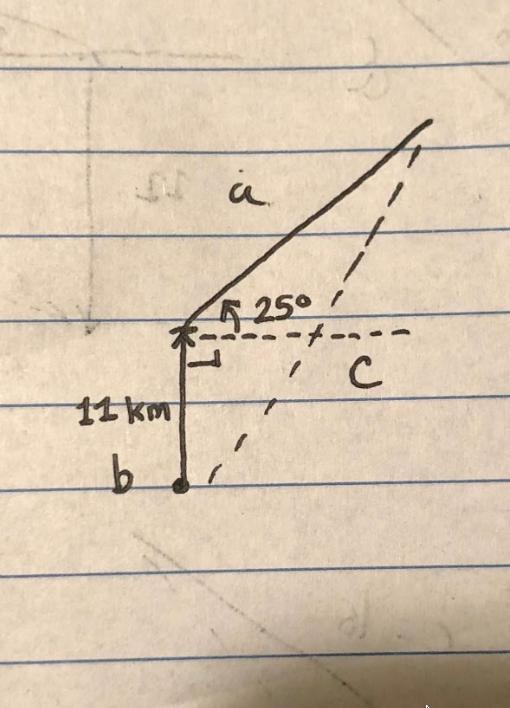Answer:
The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.The final outcome impact of the Civil War was that the North had won the war and slavery was abolished. The impact of the Civil War was the evolution of new war weapons and changes in the economy and the way people lived.
Step-by-step explanation: