Answer:
1. See the attached tree diagram (64 different sequences); 2. 64 codons; 3. 8 codons; 4. 24 codons consist of three different bases.
Step-by-step explanation:
The main thing to solve this kind of problem, it is to know if the pool of elements admits <em>repetition</em> and if the <em>order matters</em> in the sequences or collections of objects that we can form.
In this problem, we have the bases of the DNA molecule, namely, adenine (A), thymine (T), guanine (G) and cytosine (C) and they may appear in a sequence of three bases (codon) more than once. In other words, <em>repetition is allowed</em>.
We can also notice that <em>order matters</em> in this problem since the position of the base in the sequence makes a difference in it, i.e. a codon (ATA) is different from codon (TAA) or (AAT).
Then, we are in front of sequences that admit repetitions and the order they may appear makes a difference on them, and the formula for this is as follows:
(1)
They are sequences of <em>k</em> objects from a pool of <em>n</em> objects where the order they may appear matters and can appeared more than once (repetition allowed).
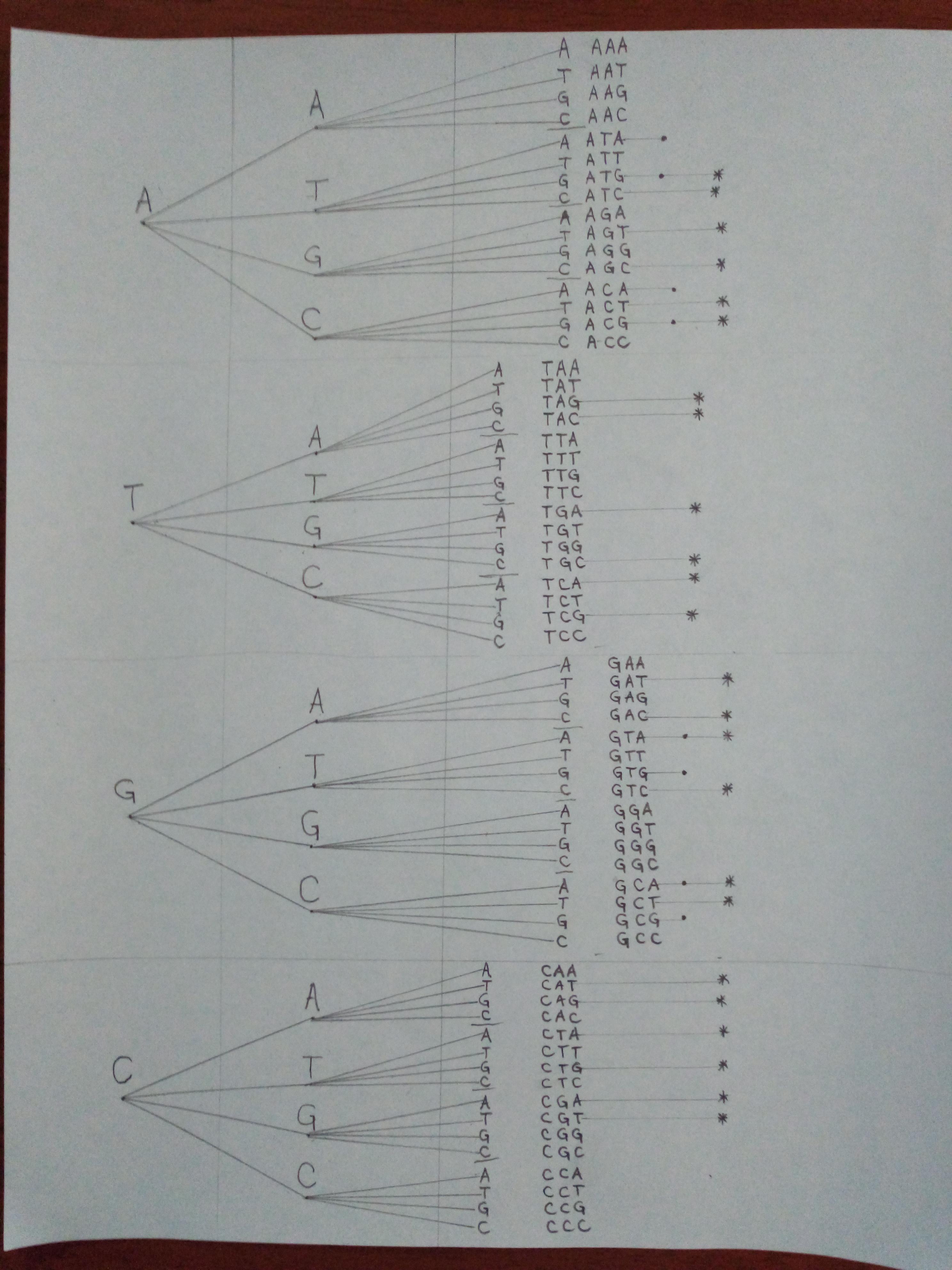
<h3>1 and 2. Possible base sequences using tree diagram and number of possible codons</h3>Having all the previous information, we can solve this question as follows:
All possible base sequences are represented in the first graph below (left graph) and are 64 since <em>n</em> = 4 and <em>k</em> = 3.
Looking at the graph there are 4 bases * 4 bases * 4 bases and they form 64 possible sequences of three bases or codons. So <em>there are 64 different codons</em>. Graphically, AAA is the first case, then AAT, the second case, and so on until complete all possible sequences. The second graph shows another method using a kind of matrices with the same results.
<h3>3. Cases for codons whose first and third bases are purines and whose second base is a pyrimidine</h3>In this case, we also have sequences with <em>repetitions</em> and the <em>order matters</em>.
So we can use the same formula (1) as before, taking into account that we need to form sequences of one object for each place (we admit only a Purine) from a pool of two objects (we have two Purines: A and G) for the <em>first place</em> of the codon. The <em>third place</em> of the codon follows the same rules to be formed.
For the <em>second place</em> of the codon, we have a similar case: we have two Pyrimidines (C and T) and we need to form sequences of one object for this second place in the codon.
Thus, mathematically:
All these sequences can be seen in the first graph (left graph) representing dots. They are:
The second graph also shows these sequences (right graph).
<h3>4. Possible codons that consist of three different bases</h3>In this case, we have different conditions: still, order matters but no repetition is allowed since the codons must consist of three different bases.
This is a case of <em>permutation</em>, and the formula for this is as follows:
(2)
Where n! is the symbol for factorial of number <em>n</em>.
In words, we need to form different sequences (order matters with no repetition) of three objects (a codon) (k = 3) from a pool of four objects (n = 4) (four bases: A, T, G, and C).
Then, the possible number of codons that consist of three different bases--using formula (2)--is:
Thus, there are <em>24 possible cases for codons that consist of three different bases</em> and are graphically displayed in both graphs (as an asterisk symbol for left graph and closed in circles in right graph).
These sequences are:
{ATG, ATC, AGT, AGC, ACT, ACG, TAG, TAC, TGA, TGC, TCA, TCG, GAT, GAC, GTA, GTC, GCA, GCT, CAT, CAG, CTA, CTG, CGA, CGT}
<h3 />