Problem 18
Answers:
~P -> Q: "if it is not fall, then the leaves change colors"
~P -> ~Q: "if it is not fall, then the leaves don't change colors"
P -> ~Q: "If it is fall, then the leaves don't change colors"
Q -> ~P: "If the leaves change colors, then it is not fall"
----------
Explanation:
Simply replace each letter with the corresponding definition. We replace each P with "it is fall" and each ~P with "it is not fall". The squiggly line means "not". The same idea applies with Q and ~Q.
==========================================
Problem 19
Answer: Choice A) 134
----------
Explanation:
AB = CD
8x = 4x+24
8x-4x = 24
4x = 24
x = 6
BC = 18x+26
BC = 18*6+26
BC = 134
==========================================
Problem 20
Answer: Choice C) x = 5 and y = 11
----------
Explanation:
(anglePQR)+(angleRQS) = 90
(12x-2)+(6x+2) = 90
18x = 90
x = 5
angle TQU = 90
10y-20 = 90
10y = 110
y = 11
==========================================
Problem 21
Answer: 99
----------
Explanation:
The remote interior angles add to the exterior angle (remote interior angle theorem)
(angle RPQ) + (angle PQR) = angle PRS
(3x-29) + (7x-42) = 6x-3
10x-71 = 6x-3
10x-6x = -3+71
4x = 68
x = 68/4
x = 17
angle PRS = 6x-3
angle PRS = 6*17-3
angle PRS = 99
==========================================
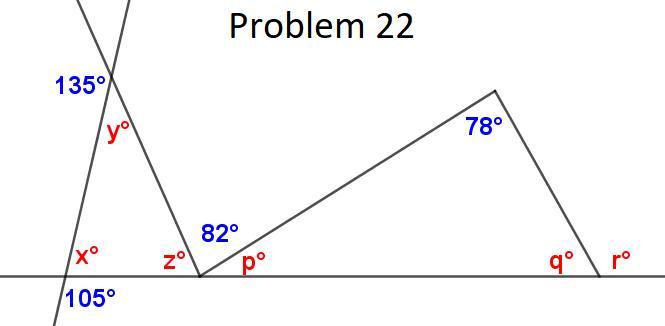
Problem 22
Answer: 116
----------
Explanation:
Refer to the diagram below.
Solve 105+x = 180 to get x = 75
Solve 135+y = 180 to get y = 45
Solve x+y+z = 180 to get z = 60
Solve z+82+p = 180 to get p = 38
Solve p+78+q = 180 to get q = 64
Solve q+r = 180 to get r = 116
==========================================
Problem 23
Answer: B) between 4 and 44 inches
----------
Explanation:
We use a variation of the triangle inequality theorem here.
If p is the missing side length, then
24-20 < p < 24+20
4 < p < 44
So,
4 < XY < 44
The side XY cannot be 4 inches and it cannot be 44 inches either.
==========================================
Problem 24
4 Answers: A, B, C, D
----------
Explanation:
Same idea as problem 23
24-10 < x < 24+10
14 < x < 34
So we'll select values between 14 and 34, excluding each endpoint. Those values are x = 16, 18, 24, 26
In other words, only 12 and 34 are non-answers.
==========================================
Problem 25
Answer: C) 84 degrees
----------
Explanation:
The given exterior angle is 152 degrees
Subtract that from 180 to get the adjacent interior angle 180-152 = 28
Now let's find the missing angle of triangle DEB
D+E+B = 180
28+68+B = 180
96+B = 180
B = 180-96
B = 84
This is the measure of angle DBE
This is also the measure of angle ABC because the two angles are vertical angles.
Vertical angles are always congruent.