Answer:
Each principal energy level above the first contains one s orbital and three p orbitals. A set of three p orbitals, called the p sublevel, can hold a maximum of six electrons. Therefore, the second level can contain a maximum of eight electrons - that is, two in the s orbital and 6 in the three p orbitals.
Explanation:
Answer:
Multiply 3 by Avogadro's number.
Explanation:
The mole can be defined as the amount of a substance that contains Avogadro’s number of particles, 6.02 x 10²³.
For elementary particles:
Number of particles=
number of moles x 6.02 x10²³
From the question,
Number of moles = 3moles
Number of particles = 3 x 6.02 x10²³
Explanation:
B. Recycles slowly
And it also depends on the organism
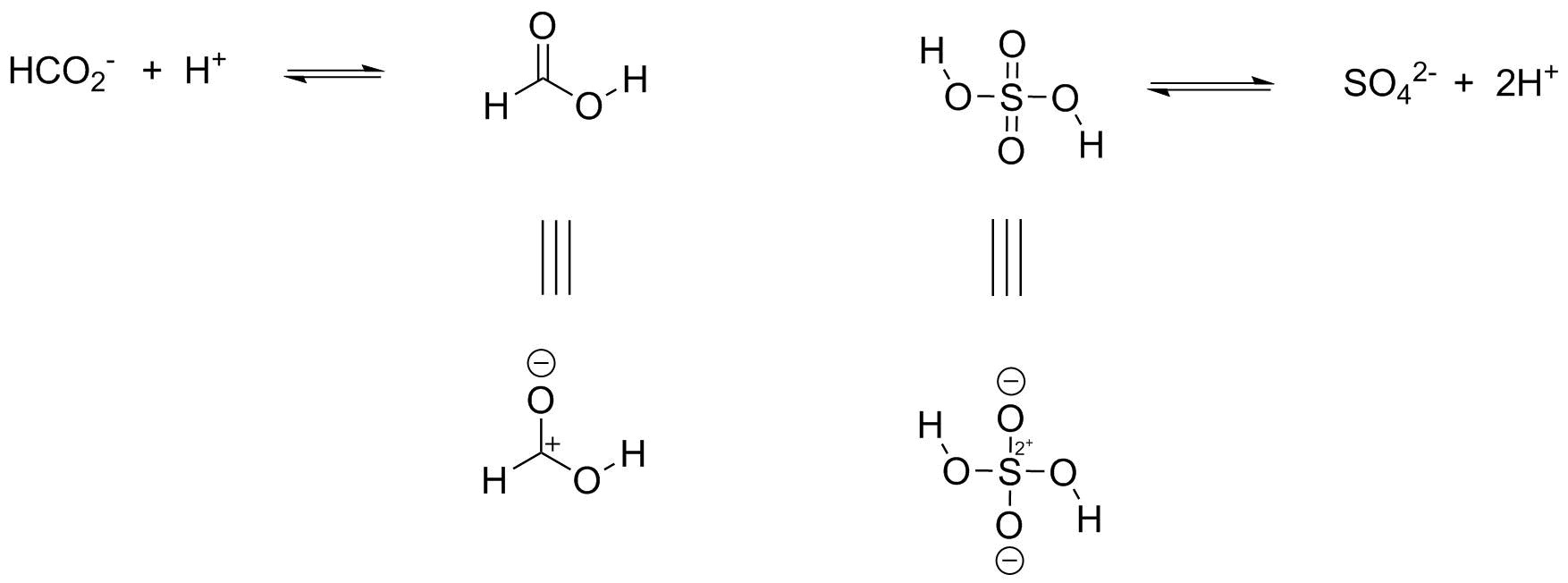
Among formic acid (HCOOH ) and sulfuric acid (H₂SO₄), formic acid is the weak acid. Acidic strength of any acid is the tendency of that acid to loose proton. Among these two acids formic acid has a pKa value of 3.74 greater than that of sulfuric acid i.e. -10. Remember! Greater the pKa value of acid weaker is that acid and vice versa. Below I have drawn the Ionization of both acids to corresponding conjugate bases and protons. The structures below with charges are drawn in order to explain the reason for strength. As it is seen in charged structure of formic acid, there is one positive charge on carbon next to oxygen carrying proton. The electron density is shifted toward carbon as it is electron deficient and demands more electron hence, attracting electron density from oxygen and making the oxygen hydrogen bond more polar. While, in case of sulfuric acid it is depicted that Sulfur attached to oxygen containing proton has 2+ charge, means more electron deficient as compared to carbon of formic acid, hence, more electron demanding and strongly attracting electrons from oxygen and making the oxygen hydrogen bond very polar and highly ionizable.
The battery gives energy to the connected light and switch, and the switch controls the light.