1) Progressive waves are the ones by which all the wave disturbances are carried on further and propelled or transferred forward.
1) Stationary waves are the ones by which all the wave disturbances are not carried on further and propelled or transferred forward.
2) In Progressive waves the energies are equally and efficiently transferred along the travelling waves. Every particle are transferring some kind of energy to a next further particle on the same path, basically most of the energies are lost because of which there's no energy acquired by it.
2) In Stationary or standing waves there's no absolute transfer of any significant amount of energies which are not transferred along a path of the wave. Particles in stationery waves are giving and contributing in energy submission and also acquire some of the energy back because of which the net transferring of energies between the particles in a specific period as nullified.
3) Phases of the progressive waves of the particles in these waves are varying in a continuous manner and have changing values between them.
3) Phases of the Stationary or standing waves of the particles in these waves are not changing and always same to the contrary opposite when placed between the consecutively running sets of nodes (Between two nodes of particles).
4) Progressive waves have no particles which show they're having a rest phase or a permanent rest phase in a medium (particle medium).
4) Stationary waves have significant amount of particles of the medium to show that there having a rest phase or a permanent rest phase at the nodes of those particles.
5) Amplitudes of Progressive waves are totally and completely different particles are neutral and are having same values.
5) Amplitudes of Stationary or Standing waves of the particles in between those tow consecutively sets of nodes in between them and antinodes provided are having different values and vary much more progressively.
6) All of the particles in Progressive waves containing it don't specially cross their given mean positions in a simultaneous manner.
6) All of the particles in Stationary waves containing it frequently and steadily cross their given mean positions in a simultaneous manner.
7) In Progressive waves the particles don't show any attainment of a displacement provided in a maximum amount in a simulations manner.
7) In Stationary waves the particles are showing and exhibiting the attainments of various displacements in a maximum amount in a simultaneous manner.
8) Maximum velocities achieved by Progressive waves are indeed same or similar for all the given particles when they're showing a passing of those given mean positions.
8) Maximum velocities achieved by Stationary waves of those particles when they're crossing their given mean positions are in a continuity of increasement for those particles between those "nodes" and of course the consecutively set "antinodes" further which it's showing a significant decreasement after it corresponds and reaches the second or usually the next nearest node.
9) Progressive waves have crest and troughs in their waves that're moving into a forward direction.
9) Stationary waves have crest and troughs in their waves that're appearing and disappearing in same positions or regions that is , not moving forward in a same direction.
Read more on Brainly.in - https://brainly.in/question/1959503#readmore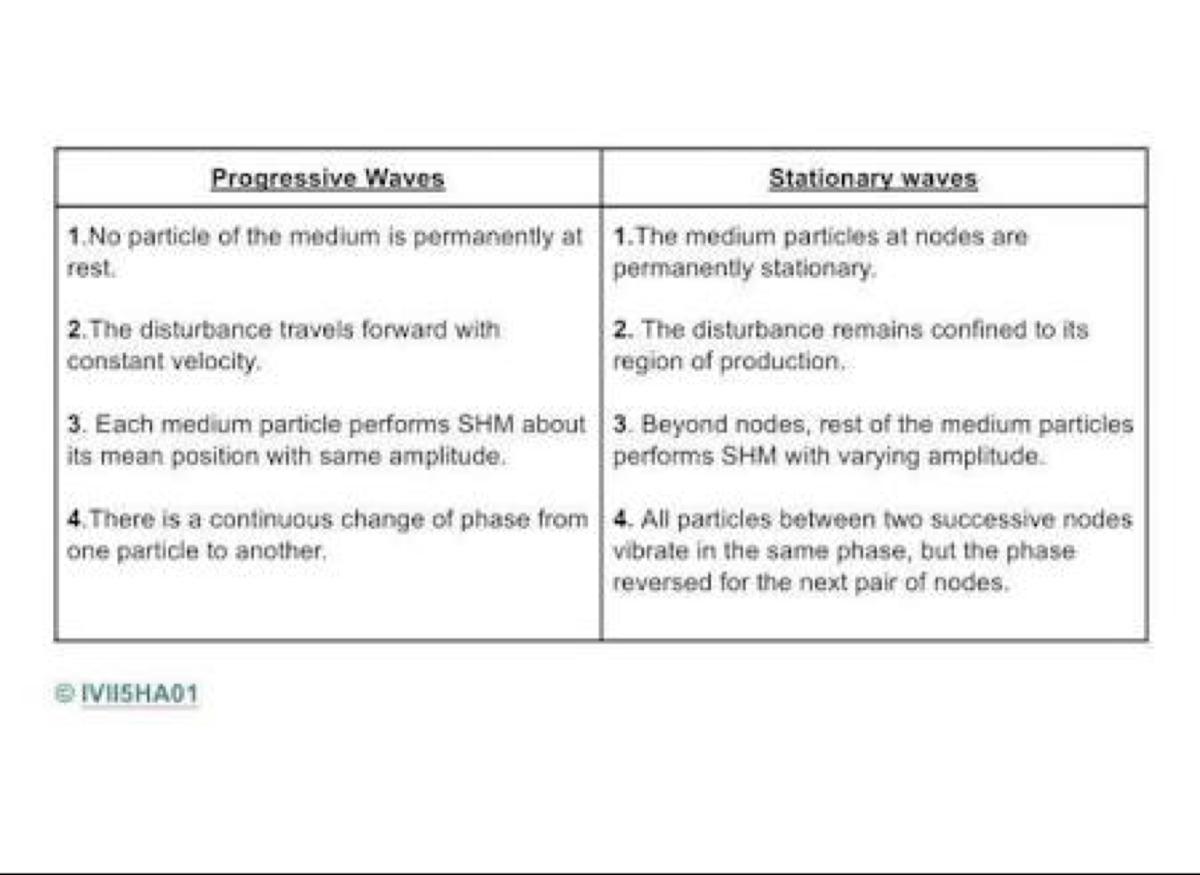
1) Stationary waves are the ones by which all the wave disturbances are not carried on further and propelled or transferred forward.
2) In Progressive waves the energies are equally and efficiently transferred along the travelling waves. Every particle are transferring some kind of energy to a next further particle on the same path, basically most of the energies are lost because of which there's no energy acquired by it.
2) In Stationary or standing waves there's no absolute transfer of any significant amount of energies which are not transferred along a path of the wave. Particles in stationery waves are giving and contributing in energy submission and also acquire some of the energy back because of which the net transferring of energies between the particles in a specific period as nullified.
3) Phases of the progressive waves of the particles in these waves are varying in a continuous manner and have changing values between them.
3) Phases of the Stationary or standing waves of the particles in these waves are not changing and always same to the contrary opposite when placed between the consecutively running sets of nodes (Between two nodes of particles).
4) Progressive waves have no particles which show they're having a rest phase or a permanent rest phase in a medium (particle medium).
4) Stationary waves have significant amount of particles of the medium to show that there having a rest phase or a permanent rest phase at the nodes of those particles.
5) Amplitudes of Progressive waves are totally and completely different particles are neutral and are having same values.
5) Amplitudes of Stationary or Standing waves of the particles in between those tow consecutively sets of nodes in between them and antinodes provided are having different values and vary much more progressively.
6) All of the particles in Progressive waves containing it don't specially cross their given mean positions in a simultaneous manner.
6) All of the particles in Stationary waves containing it frequently and steadily cross their given mean positions in a simultaneous manner.
7) In Progressive waves the particles don't show any attainment of a displacement provided in a maximum amount in a simulations manner.
7) In Stationary waves the particles are showing and exhibiting the attainments of various displacements in a maximum amount in a simultaneous manner.
8) Maximum velocities achieved by Progressive waves are indeed same or similar for all the given particles when they're showing a passing of those given mean positions.
8) Maximum velocities achieved by Stationary waves of those particles when they're crossing their given mean positions are in a continuity of increasement for those particles between those "nodes" and of course the consecutively set "antinodes" further which it's showing a significant decreasement after it corresponds and reaches the second or usually the next nearest node.
9) Progressive waves have crest and troughs in their waves that're moving into a forward direction.
9) Stationary waves have crest and troughs in their waves that're appearing and disappearing in same positions or regions that is , not moving forward in a same direction.
Read more on Brainly.in - https://brainly.in/question/1959503#readmore

7
0